Table of Contents
Scalar vs. Vector Quantity: What Is The Difference Between Scalar And Vector Quantity?
As science and mathematics bear witness to an ever-expanding realm of knowledge and development, it is important to differentiate between scalar and vector quantities. The physical world is rife with various quantities ranging from simple measurements such as length, mass, and time to the more complex forms of velocity, momentum, and force. There are essentially two types of quantities, scalar and vector. Despite the fact that the distinction between scalar and vector quantities may seem trivial, there is a clear distinction between the two that is relevant in our day to day lives, especially when dealing with complex mathematical calculations and physical phenomena. In this blog post, we will delve deeper into the differences between scalar and vector quantities, examining their definitions, examples, and the various applications of each.
What Is Scalar?

Scalar refers to a mathematical quantity that has only magnitude and no direction. In simpler terms, scalar is a physical quantity that can be measured and expressed through a single numerical value, without any additional information about where it came from or in which direction it is moving. Scalar quantities are represented by a simple number, unit of measurement, and dimensional analysis. Examples of scalar quantities include distance, time, mass, speed, temperature, and energy. Understanding scalars is essential to many fields of science, including physics, chemistry, and engineering, as it lays the foundation for the analysis of complex mathematical concepts and calculations.
What Is Vector Quantity?

A vector quantity is a physical quantity that encompasses both magnitude and direction. This means that any vector quantity cannot be explained by a single numerical value alone as it requires both the magnitude and direction of the quantity. For instance, velocity, momentum, and force are all examples of vector quantities in physics. While scalar quantities are represented only by their magnitude or numerical value, vector quantities must be depicted by both their magnitude and direction in order to be fully understood. Vector quantities play a crucial role in physics as they provide a useful framework for explaining and analyzing a range of physical phenomena. Thus, an understanding of vector quantities is essential for anyone pursuing a career or interest in physics.
Key Differences Between Scalar And Vector Quantity
Scalar and vector quantities are two essential concepts in physics that are crucial for accurately describing and measuring physical phenomena. Scalar quantities are those that possess only magnitude or size, without any reference to direction, such as length, mass, temperature, and speed. On the other hand, vector quantities are those that encompass both magnitude and direction, such as velocity, acceleration, force, and momentum. The primary difference between scalar and vector quantities is that scalar quantities can be added and subtracted algebraically, while vector quantities must take into account both magnitude and direction. Additionally, scalar quantities can be multiplied by scalar numbers, while vector quantities can be multiplied by scalar numbers as well as added or subtracted vectors. Understanding the difference between scalar and vector quantities is fundamental for solving problems in physics accurately.
- Scalar quantities are those that possess only magnitude or size, without any reference to direction.
- Vector quantities are those that encompass both magnitude and direction, such as velocity, acceleration, force, and momentum.
- Scalar quantities can be multiplied by scalar numbers, while vector quantities can be multiplied by scalar numbers as well as added or subtracted vectors.
Scalar vs. Vector Quantity Similarities
Scalar and vector quantities are both important concepts in physics and mathematics. While scalar quantity refers to measurement having only magnitude, vector quantity includes both magnitude and direction. Both these quantities are used to describe the physical world, where scalar represents the total length, area or volume, and vector represents motion in a particular direction. The similarities between them are that they both serve specific purposes in physical calculations and can express physical phenomena. Another similarity between scalar and vector quantities is that they are both represented by numerical values. The units of measurement of both these entities are also similar, since many physical quantities can be measured in mass, length, and time units.
- Both these quantities are used to describe the physical world.
- The similarities between them are that they both serve specific purposes in physical calculations and can express physical phenomena.
- Another similarity between scalar and vector quantities is that they are both represented by numerical values.
Scalar vs. Vector Quantity Pros and Cons
Scalar Pros & Cons
Scalar Pros
Scalar quantities have a significant advantage over vector quantities in physics, where vector quantities have both magnitude and direction. Scalar quantities are much simpler to understand and work with, as they only have magnitude and no direction. This makes computations easier and quicker since no vector addition is necessary. The common examples of scalar quantities are length, mass, temperature, time, and speed. Scalars can save time and reduce complexity in the analysis of various physical phenomena. Scalars can also be used in modeling and simulations, where complex vector mathematics would take up considerable resource cost. Additionally, scalar quantities simplify the interpretation of data, and their use can enhance the accuracy and reliability of measurements.
- Scalar quantities are much simpler to understand and work with, as they only have magnitude and no direction.
- Scalars can save time and reduce complexity in the analysis of various physical phenomena.
- Scalars can also be used in modeling and simulations, where complex vector mathematics would take up considerable resource cost.
Scalar Cons
Scalars have many disadvantages that make them less useful in certain circumstances. First, scalars cannot capture direction or orientation, making them unsuitable for describing many phenomena, such as vector quantities like force, torque, or velocity. Additionally, scalars cannot provide information about the relative position or orientation of objects, which limits their usefulness in fields like engineering and physics. Another disadvantage of scalars is that they cannot be added or subtracted like vectors, which can complicate calculations and make them less intuitive. Finally, some scalar quantities may be affected by contextual factors or external forces, which can make them less reliable or consistent over time. Despite these limitations, scalar quantities remain important in many areas of science, engineering, and everyday life.
- Scalars cannot capture direction or orientation, making them unsuitable for describing many phenomena.
- Scalars cannot provide information about the relative position or orientation of objects, which limits their usefulness in fields like engineering and physics.
- They cannot be added or subtracted like vectors, which can complicate calculations and make them less intuitive.
Vector Quantity Pros & Cons

Vector Quantity Pros
Vector quantity is a fundamental concept in physics where physical properties have both magnitude and direction. Advantages of vector quantity include the ability to represent complex physical phenomena in a more accurate and efficient manner. Vectors are essential in describing the motion and forces acting on particles in mechanics, and their directionality helps in determining the net forces that can bring balance. Furthermore, vector quantities can be easily manipulated and simplified through various algebraic operations such as addition, subtraction, and scalar multiplication. This makes vector analysis easier to perform and is widely applicable in numerous fields such as engineering, navigation, and space science.
- Advantages of vector quantity include the ability to represent complex physical phenomena in a more accurate and efficient manner.
- Vectors are essential in describing the motion and forces acting on particles in mechanics, and their directionality helps in determining the net forces that can bring balance.
- Vector quantities can be easily manipulated and simplified through various algebraic operations.
Vector Quantity Cons
While vector quantities possess numerous advantages in the field of physics, they inevitably have some significant drawbacks. One of the primary cons of vector quantities is their complexity. As a result of their dual nature, they require intricate computations and illustrations, which can increase the risk of human error. Furthermore, vector quantities require specialized apparatus and software to analyze and interpret, making them more expensive to work with. Additionally, vector quantities’ sensitivity to environmental factors such as temperature, air resistance, and other interferences may affect their accuracy, making them less suitable for certain applications. Finally, vector quantities’ directionality can be challenging to comprehend, making them difficult to explain to non-experts.
- One of the primary cons of vector quantities is their complexity.
- As a result of their dual nature, they require intricate computations and illustrations, which can increase the risk of human error.
- Vector quantities require specialized apparatus and software to analyze and interpret, making them more expensive to work with.
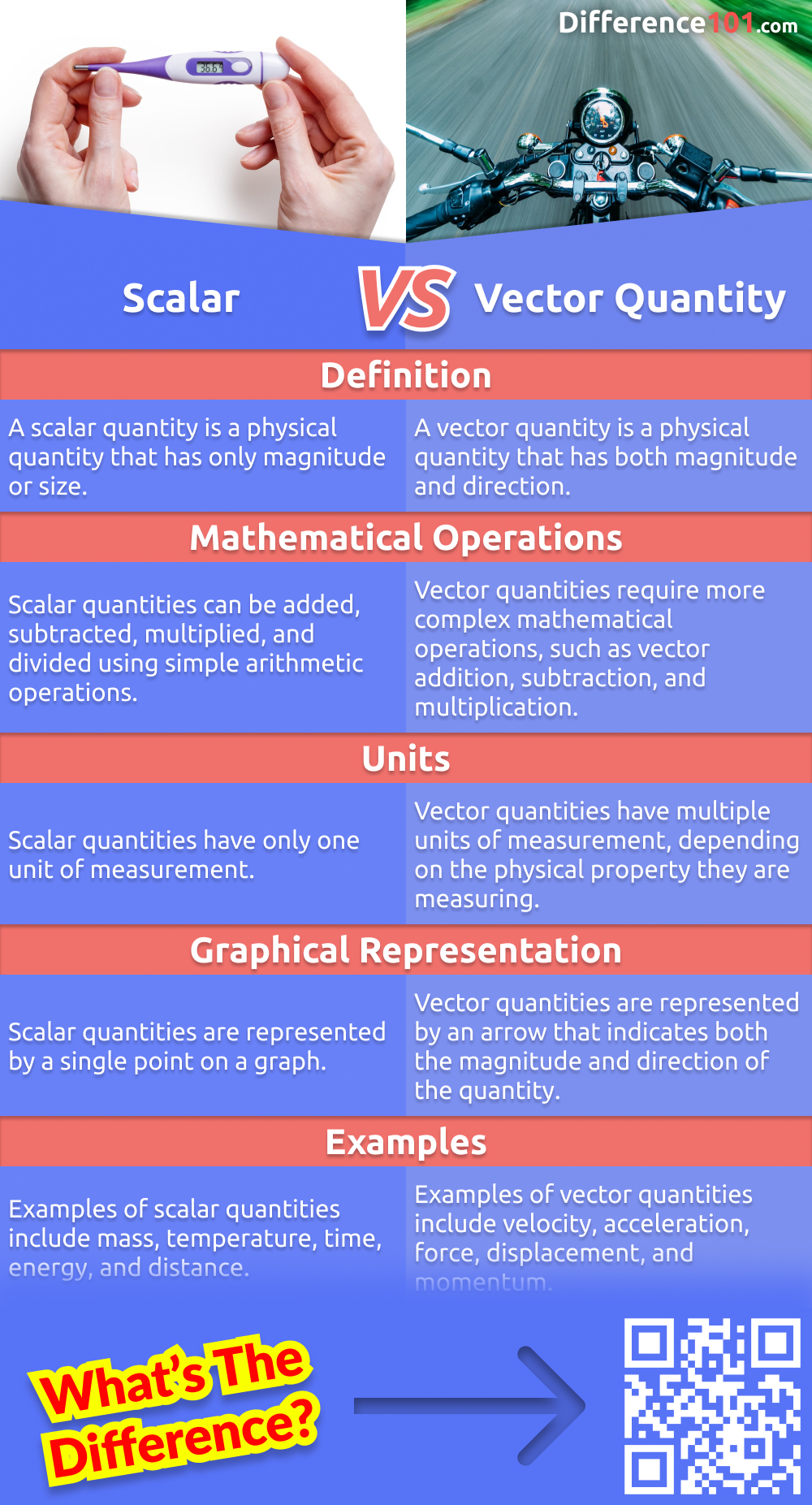
Comparison Table: 5 Key Differences Between Scalar And Vector Quantity
| Components | Scalar | Vector Quantity |
|---|---|---|
| Definition | A scalar quantity is a physical quantity that has only magnitude or size. | A vector quantity is a physical quantity that has both magnitude and direction. |
| Mathematical Operations | Scalar quantities can be added, subtracted, multiplied, and divided using simple arithmetic operations. | Vector quantities require more complex mathematical operations, such as vector addition, subtraction, and multiplication. |
| Units | Scalar quantities have only one unit of measurement. | Vector quantities have multiple units of measurement, depending on the physical property they are measuring. |
| Graphical Representation | Scalar quantities are represented by a single point on a graph. | Vector quantities are represented by an arrow that indicates both the magnitude and direction of the quantity. |
| Examples | Examples of scalar quantities include mass, temperature, time, energy, and distance. | Examples of vector quantities include velocity, acceleration, force, displacement, and momentum. |
Comparison Chart
Comparison Video
Conclusion
To sum it up, scalar and vector quantities are two fundamental concepts of physics. Scalar quantities represent the measurement of magnitude while vector quantities represent both the magnitude and direction of physical quantities. Understanding the difference between scalar and vector quantities is crucial for students of physics and their application in real-life scenarios. It is also worth noting that having a clear understanding of these concepts lays the foundation for advanced physics as well as engineering and technical fields.







